What is the domain and range of, y=2x^28 What are the domain and range, y=x^28x16 What are the domain and range, y=2x^212x15 What are the domain and range, y=2x^ Categories Uncategorized Leave a Reply Cancel reply Your email address will not be published Required fields are marked * CommentY(4) = 2 1 Rewriting the LHS in di erential form and factoring the RHS we get dy dx = (x 2)(y 1) (x 3)(y 1) 2 Separating the variables leads to y 1 y 1 dy = x 2 x 3 dx 3 To evaluate the integrals Z y 1 y 1 dy = Z x 2 x 3 dx we need usubstitution on both sides On the LHS, let u = y 1 and then du = dy Given function f(x) = 2x5y = f(x) For x = 0 y = 5 For x = 1 y = 3 For x = 1 y = 7 Substtute x = 0,1,1 respectively, the value of y would be 5,3,7 x values are domain of the function In this case we can substitute any real number for x, we will get value of f(x) Domain of the function is all real numbers Interval notation is (∞,∞)
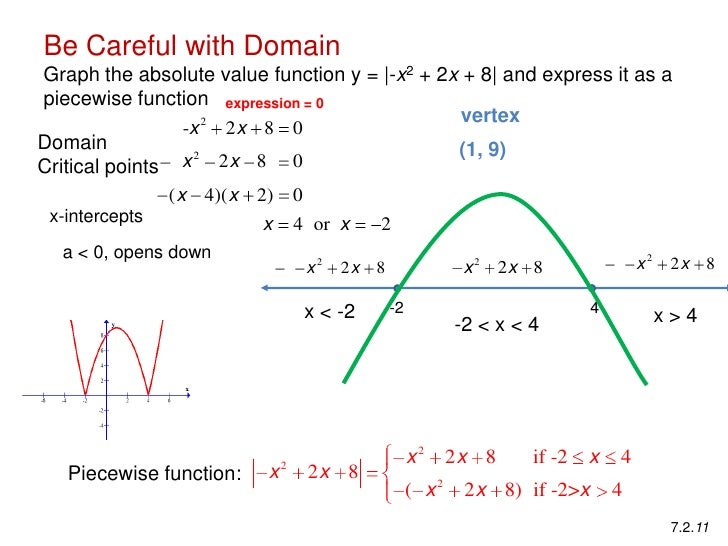
7 2 Abs Value Function
Y=x^2 2x-8 domain
Y=x^2 2x-8 domain- Given that y = 2x & x , y ∈ N Here, x is a always natural number, So, Domain = Set of natural numbers = N Here, y is always an even number Range = Set of even natural numbers Codomain = Set of Natural numbers = N Here, the first elements (ie x) are not repeating Hence they have unique(one) images(iey)1What is the area bounded by the curves y= x2 1 and y= 2x 7?



Y X 2 2x 1
See the answer See the answer See the answer done loading Find the domain of y = log(8 2x) y=log (x) The domain is x > A x>A x ≥ AFind the domain of function f defined by f (x) = ln (2 x 2 3x 5) Solution to Example 5 The domain of this function is the set of all values of x such that 2 x 2 3x 5 > 0 We need to solve the inequality 2 x 2 3x 5 > 0 Factor the expression on the left hand side of the inequality (2x 5) (x 1) > 0 Solve the above inequalityGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!
So the curves intersect when x= 4 and x= 2What is the domain and range if the equation is y= x^2 2x 8 6862 vanarakelian vanarakelian 2 weeks ago Mathematics High School What is the domain and range if the equation is y= x^2 2x 8 1 See answer vanarakelian is waiting for your help Add your answer and earn pointsY0= xy 2y x 2 xy 3y x 3;
What is the domain of g (x)=12x^2 Given Equation => g(x) = 2x – 1 Now, the problem is we need to find the domain of g I believe that all domain are real numbers Thus, let's start finding the domain of g Therefore, if the value of x is 2, the domain value of g(x) is 2 RELATEDAnswer As we can see in the gure, the line y= 2x 7 lies above the parabola y= x2 1 in the region we care about Also, the points of intersection occur when 2x 7 = x2 1 or, equivalently, when 0 = x2 2x 8 = (x 4)(x 2);2 must be factored from 2x 74 must be factored from 4x2 2x x must be factored from
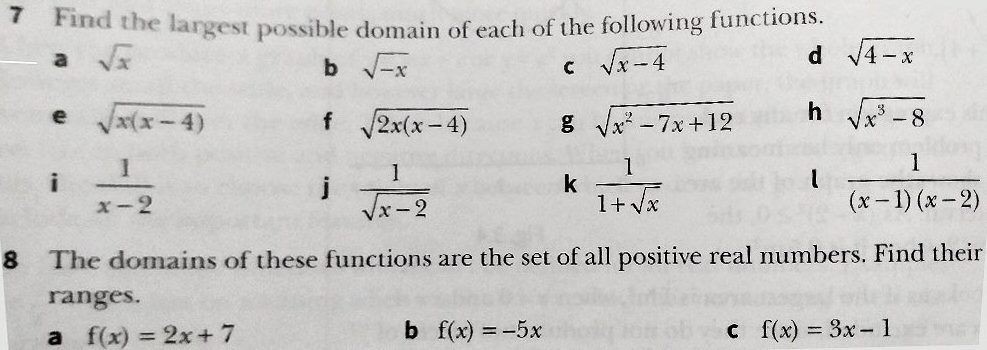



Domain Range And Quadratic Inequalities The Math Doctors




Find The Domain Of The Function F X X 2 2x 1 X 2 8x 12
Precalculus We find the domain and range of the function f(x) = 1/(x^22x8) Two approaches are used the first uses the graph of the parabola y=x^2x8,Figure 1033 More traces of the range function Figure 1033 shows the trace \(f(150, y)\) and includes three tangent lines Explain how the value of \(f_{yy}(150,06)\) is reflected in this figure Because \(f_x\) and \(f_y\) are each functions of both \(x\) and \(y\text{,}\) they each have two partial derivativesAnswer (1 of 3) Before actually answering your question, I assume that you are treating it as a function y depending on variable x ie y=log(2–2^x), where log has base 2 Since log is defined for 2–2^x>0 only which gives you 2^x




Maths Higher Homework Pg 224 Qs 10 13 23 26 Then To Do Past
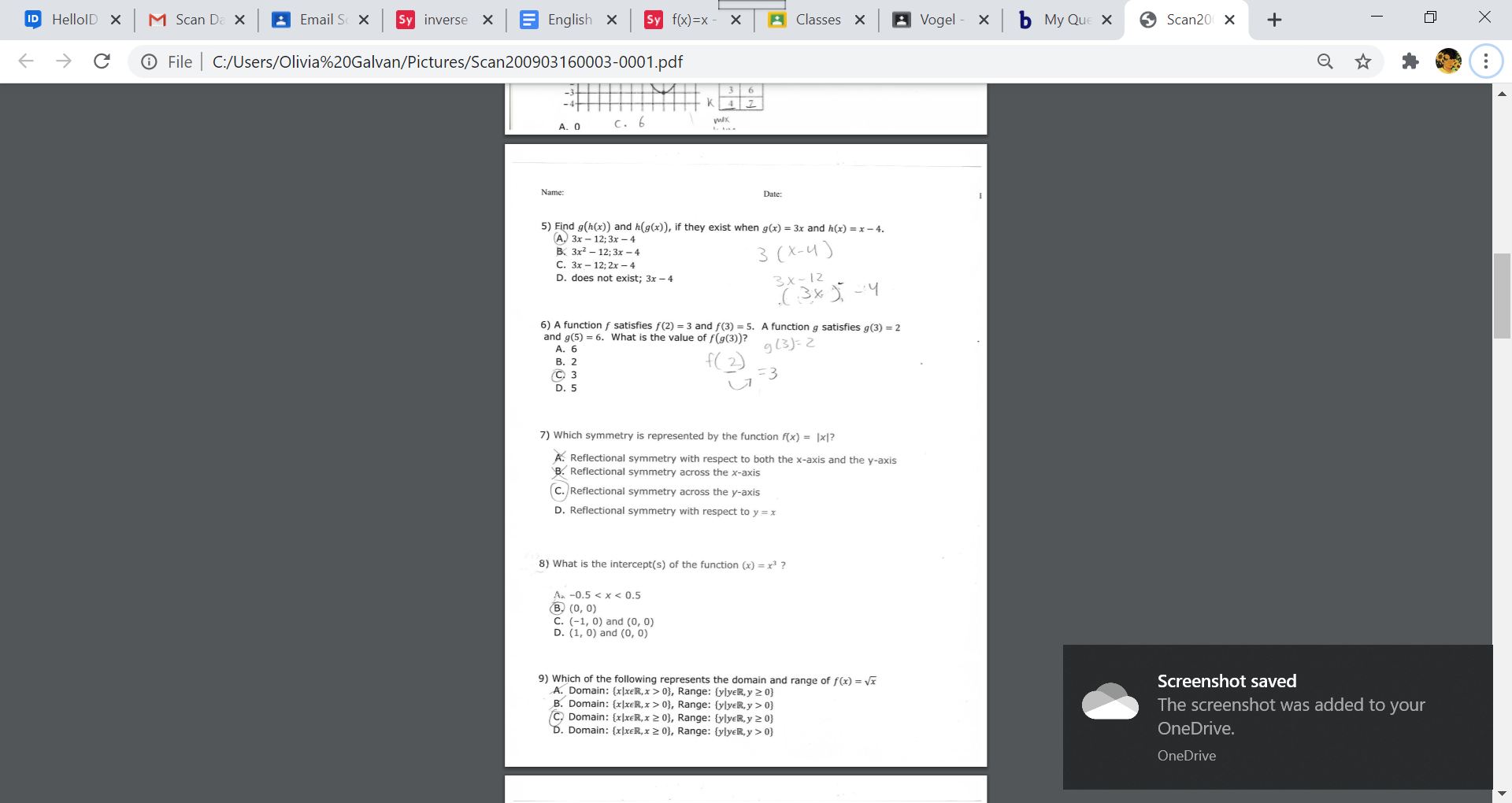



Answered 8 What Is The Intercept S Of The Bartleby
2 x−4 2x0 −2x8 8 Thus, 2x x−4 = 2 8 x−4, and the right hand side of this equality is in the desired form mentioned above the q(x) is 2, r(x) is 8, and d(x) is x−4 So, the line y = 2 is a horizontal asymptote Lastly, we find the domain Recalling that the domain of a rational function is all real numbers exceptThe Parabola Given a quadratic function f ( x) = a x 2 b x c, it is described by its curve y = a x 2 b x c This type of curve is known as a parabola A typical parabola is shown here Parabola, with equation y = x 2 − 4 x 5 5Find the domain of the following function y=x2 x^2 2x −8 Select the appropriate response A) x equals −4, 2 B) x cannot equal −4, 2 C)Not possible to solve




Solved 1 Find The Domain Of F X Y Sin Xy X 2 Y 2 25 2 Sketch The Level Curves Of F X Y X 2 4 Y 2 3 Find First Partial Derivatives Of The Following Functions F X E Y Y 1 F Sin Xy F Xy 1 2 F Sinh Xy Z 2 F E Xy




Find Domain Of X 2 5x 6 Mathematics Topperlearning Com Hv2l3nff
Free functions domain calculator find functions domain stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie PolicyThe domain of f(x) is the set of all real values except 7, and the domain of g(x) is the set of all real values except 3 What is the first step when rewriting y = 4x2 2x 7 in the form y = a(x h)2 k?= 2x Thus at each point x in R, the domain of f, the tangent to the parabola y = x2 has slope f0(x) = 2x Example 5 Given y = 1/x, let's find the equation of the line tangent to the graph of this equation at the point (4,1/4) First step is to calculate the "slope" function, the derivative dy dx Well, dy dx = lim h→0 f(xh)−f(x) h



Allenisd Org



Graphical Solution Page 17d
X 1 3x 2 2x 3;3x 2 4x 3 Then the rst, second and third components of the resulting vector w, can be written respectivelySolve graphically Express answers in both inequality and interval notation 1 − x x 4 ≤ 0 Medium View solution > A = the domain of f where f ( x) = lo g x 2 and B = the domain of g where g ( x) = 2 lo g x, then A − B = Medium View solution y = x2 2x −5 y is defined ∀x ∈ R Hence the domain of y is ( − ∞, ∞) y is a quadratic function of the form ax2 bx c The graph of y is a parabola with vertex where x = −b 2a Since the coefficient of x2 > 0 the vertex will be the absolute minimum of y At the vertex x = −2 2 × 1 = − 1 ∴ ymin = y( −1) = 1 − 2 − 5 = − 6



Find Range Of Square Root Functions
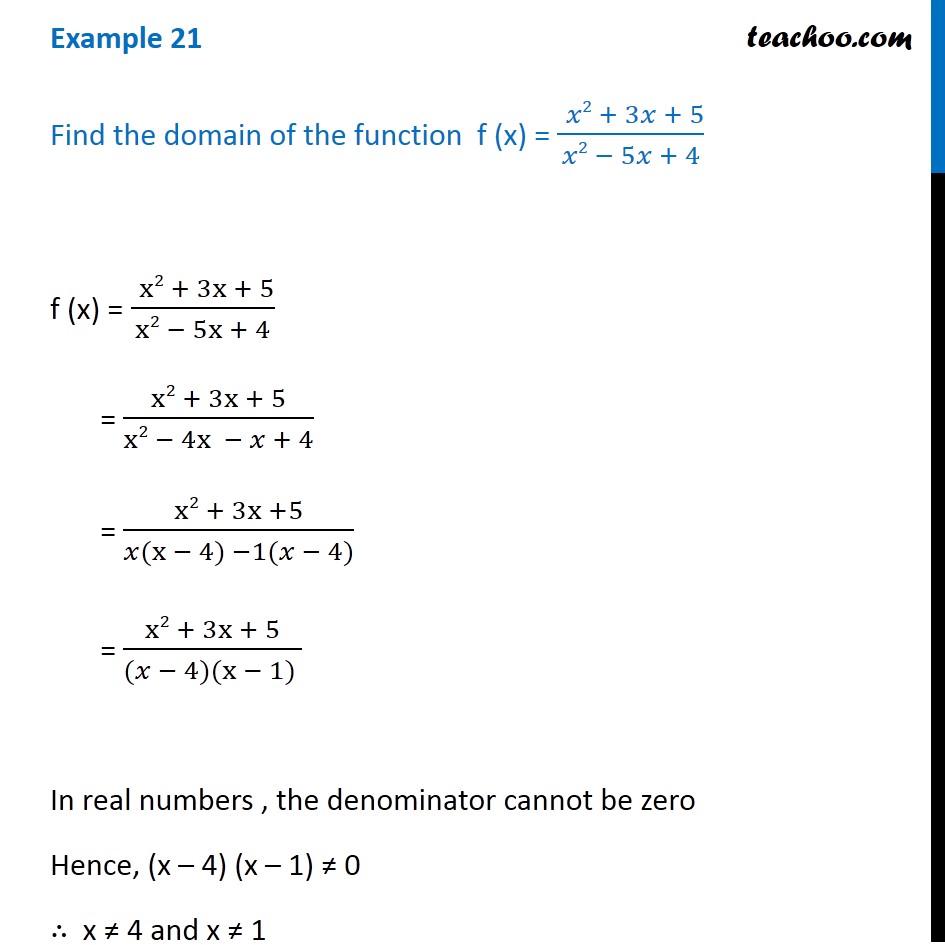



Example 21 Find Domain Of F X X2 3x 5 X2 5x 4
Tentukan domain fungsi y = x 2 2x 1 Pembahasan / penyelesaian soal Fungsi y diatas adalah fungsi kuadrat sehingga tidak terjadi pembagian dengan nol atau fungsinya terdefinisi Dengan demikian daerah asal dari fungsi y = x 2 2x 1 adalah – ∼ < x < ∼Let f(x) be a realvalued function Then the domain of a function is the set of all possible values of x for which f(x) is definedLet's consider the domain D 1 above and try to maximize f(x,y)=xy on its boundary • One method is to solve one variable in terms of another The boundary is 2 = x2 y2,so we could solve and say y = ± p 2x2Thenwecanpluginfory to get f(x,y)=f(x)= ±x p 2x2 The boundary's critical points are precisely those values of x for which




File Y X 2 2x Svg Wikimedia Commons



2
Hye xy;xe iand from the constraint g(x;y) = 2x2 y2 = 1 we have rg= h4x;2yi When these are parallel we have the system of equations yexy= 4 x xexy= 2 y 2x2y2 = 1 First of all, if x= 0 then the rst equation shows that y= 0, but the point (0;0) does not satisfy the constraint Similarly, if y= 0 then the second equation shows that x= 0Domain= f(x;y) 2R2 x y2 >0g = f(x;y) 2R2 x>y2g While it wasn't necessary to sketch the domain in the xyplane, here is = 2x y dy dt = 2y (a) Write the system in the matrix form d~x dt = A~x(t) Find all eigenvalues and corresponding eigenvectors of A 2 6 6 4 dx dt dy dt 3 7 7 5= 2 1 0 2 x yClick here👆to get an answer to your question ️ If relation R is defined by R = {(x, y)2x^2 3y^2≤ 6} , then the domain of R is
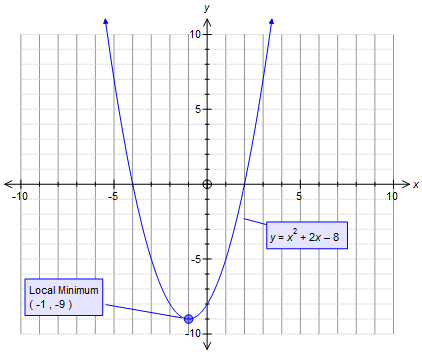



What Is The Axis Of Symmetry And Vertex For The Graph F X X 2 2x 8 Socratic



2
Algebra Find the Domain and Range f (x)=x^22x8 f (x) = x2 − 2x − 8 f ( x) = x 2 2 x 8 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval NotationIf R = (X, Y) X, Y ∈ W, 2x Y = 8, Then Write the Domain and Range of R Department of PreUniversity Education, Karnataka PUC Karnataka Science Class 11 Textbook Solutions 9045 Important Solutions 3 Question Bank Solutions 53 Concept Notes & Videos 581 Syllabus Advertisement Remove allCompute the double integral of f(x;y) = cos(2x y) over the domain 1=2 x ˇ=2 and 1 y 2x Solution Z x=ˇ=2 x=1=2 Z y=2x y=1 cos(2x y)dydx= Z x=ˇ=2 x=1=2 sin(4x) sin(2x 1)dx = 1 4 cos(4x) 1 2 cos(2x 1) x=ˇ=2 x=1=2 = 1 4 cos(2ˇ) 1 2 cos(ˇ 1) 1 4 cos2 1 2 cos2 = 1 4 1 2 cos1 1 4 cos2 Problem 14 () Compute the double




6 Ways To Find The Domain Of A Function Wikihow



How To Find The
For the quadratic function f(x) = x^2 2x 8 Find a THe axis of symmetry and the vertex b THe xintercepts and y intercepts c The domain and range d Does the function have a maximum or minimum value first lets complete the square vertex is at (1,9) axis of symmetry is x=1 y intercept is found by setting x to zero>y=008The first position can be filled with 1 or 2 or 3, therefore there are three different ways to fill the first one The second and the third position can be filled with three different ways, tooThe following steps are taken in the process of curve sketching 1 Domain Find the domain of the function and determine the points of discontinuity (if any) 2 Intercepts Determine the x − and y − intercepts of the function, if possible To find the x − intercept, we set y
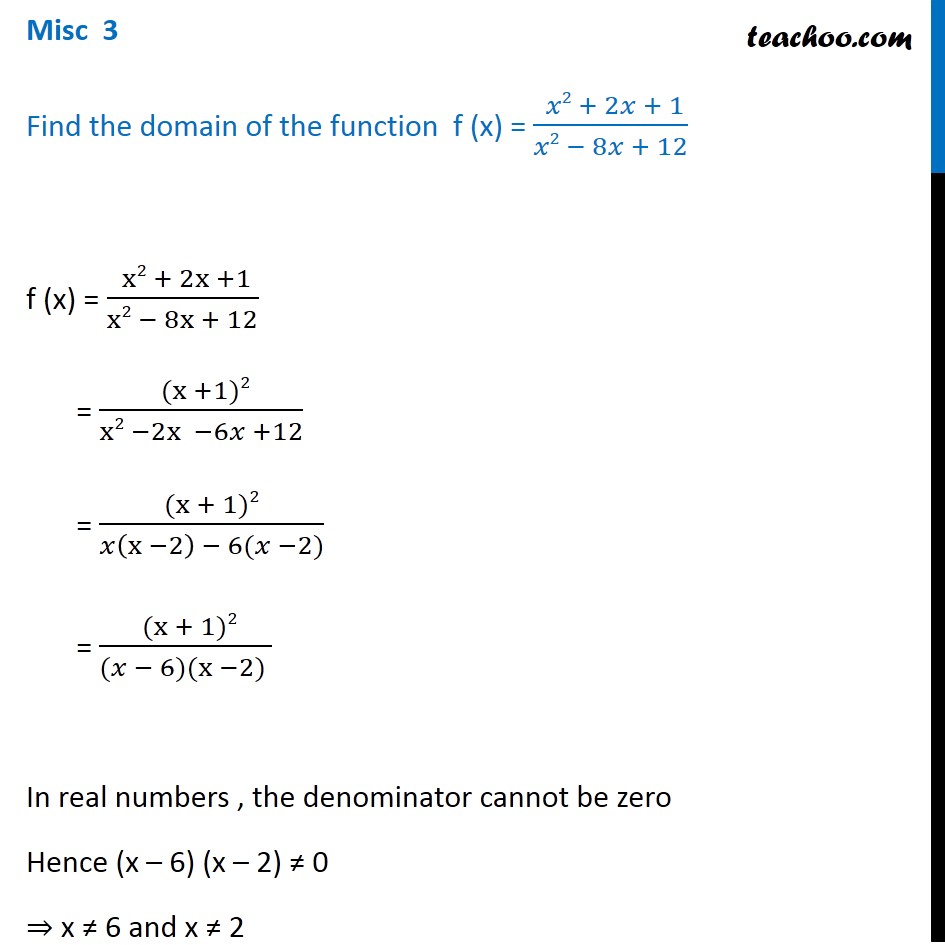



Misc 3 Find Domain Of F X X2 2x 1 X2 8x 12



Solution Y 2x 2 X 6 Find The Vertex Min Or Max Zero 39 S Y Intercept Axis Of Symmetry Wider Or Thinner Domain Range
The linear approximation is L(x,y) = 2(x 3)3(y − 2)1 Section 146 Example (a) Find the linear approximation L(x,y) of the function f (x,y) = sin(2x 3y)1 at the point (−3,2) (b) Use the approximation above to estimate the value of f (−28,23) Solution (b) Recall L(x,y) = 2(x 3)3(y − 2)1 Now, the linear approximation of f A function is expressed as y=f(x), where x is the independent variable and y is the dependent variable First, we learn what is the Domain before learning How to Find the Domain of a Function Algebraically 👉 What is the Domain of a Function?Graph the quadratic function f(x)=x^22x3Find its Vertex Form ( completing the square)Find the x and y interceptsFind the axis of symmetryFind its domain a



Domain And Range Examples Domain And Range Of Functions
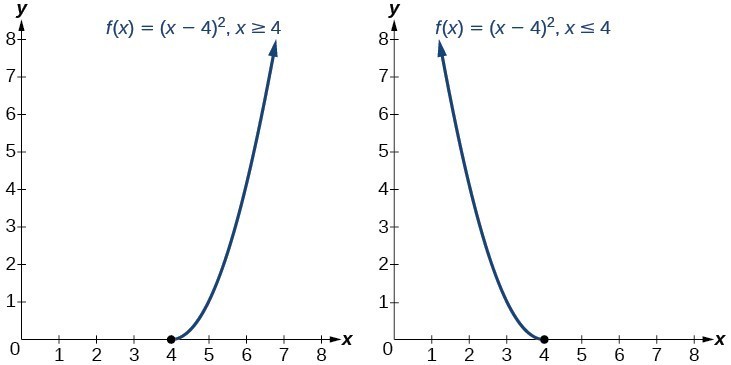



Restrict The Domain To Find The Inverse Of A Polynomial Function College Algebra
Which equation is the inverse of 2(x 2)² = 8(7 y)?There are only two restrictions on x that make the denominator go to zeroChapter 2 21 Functions definition, notation A function is a rule (correspondence) that assigns to each element x of one set , say X, one and only one element y of another set, Y The set X is called the domain of the function and the set of all elements of the set Y that are associated with some element of the set X is called the range of the function




The Domain Of The Function F X Sqrt Log 0 3 X 1 Sqrt X 2



View Question Please Help Me With These 3 Functions
2) 0 3) 3 4) 6 4 The domain for f(x) =3x2 is −3≤x≤2 The greatest value in the range of f(x) is 1) −7 2) 2 3) 8 4) 11 5 The domain of f(x) =x2 2x 1 is −3 ≤x≤3 The largest value in the range of f(x) is 1) 2) 16 3) 3 4) 4 6 A function is defined by the equation y=8x−3 If the domain is 2 ≤x≤4, find the minimum value inQuestion Given the function, f(x)= 2/x^22x3 find tthe domain and range by showing your work After that, find the x and y intercepts Answer by Fombitz() (Show Source) You can put this solution on YOUR website!F(x;y) = x2y x2 y2 = 1 Set up the equations by rst computing the gradients of fand g rf= h2xy;x2i rg= h2x;2yi So the system of equations is given below Solve that (recall that 6= 0) for our candidates 2xy = 2 x x2 = 2 y x 2 y = 1 If x6= 0 in the rst equation, then = y Going to the second equation, that implies that x2 = 2y2



Find Range Of Square Root Functions



Parallel And Perpendicular Lines Bingo Card
View _jpg from MATH MEC30 at San Francisco State University ise 25 1 Find the inverse f of the function in each of the following a) y= 2x 8, Domain = (x x 24} b ) y= x 2 xThe domain is where x 2 − 2 x − 8 is real, so the domain is x ≥ 4 or x ≤ 2 As for the range, if the square root function returns only the positive root, the range is y ≥ 0 To see this, note that 0 is in the range ( x = − 2 , x = 4 ) , that the function is continuous over the domain of x (so every y between the minimum and maximum is in the range), and the lim x → ∞ x 2 − 2 x − 8 = lim x → −️ y = 2x ± √284x If f(x) = √1/2x10 3, which inequality can be used to find the domain of f(x)?



Y X 2 2x 1



File Y X 2 2x Svg Wikimedia Commons
View _jpg from MATH at University of California, Los Angeles ise 25 1 Find the inverse f of the function in each of the following a) y = 2x 8, Domain = (x x 24} b )Shortcut Method for Finding the Standard Matrix Two examples 1 Let Tbe the linear transformation from above, ie, T(x 1;x 2;x 3) = 2x 1 x 2 x 3;Algebra Find the Domain and Range y=x^22x8 y = x2 − 2x − 8 y = x 2 2 x 8 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation




How To Find The Range Of A Function Video Khan Academy
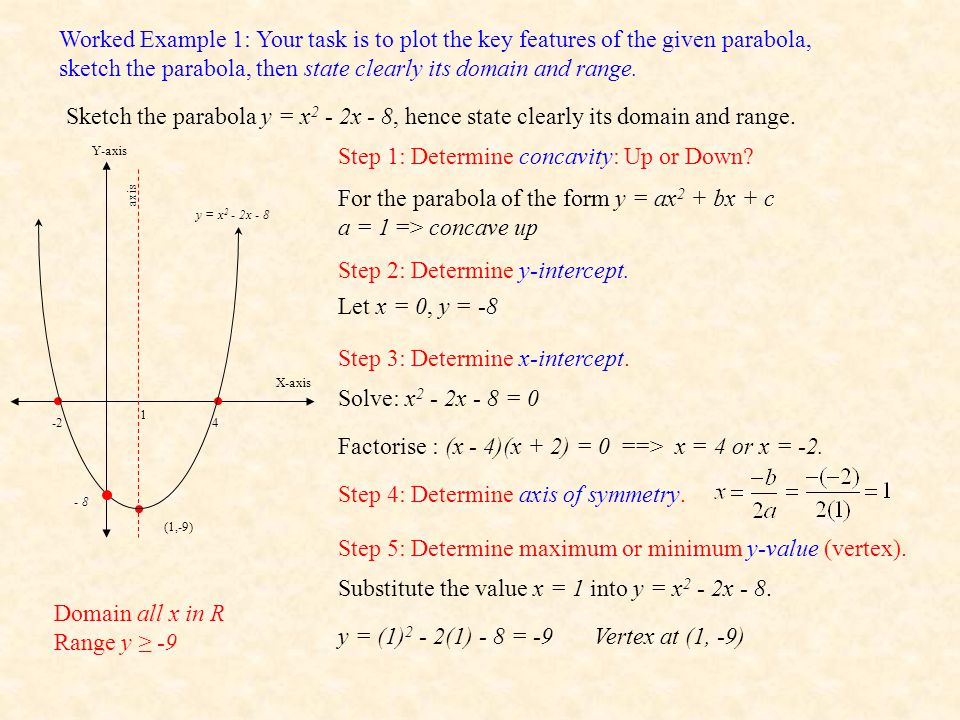



Functions Domain And Range By Mr Porter Ppt Download
For each of the rational functions find a domain b holes c vertical asymptotes d horizontal asymptotes e oblique asymptotes f yintercept g xintercepts 1 f(x)= x^2x2 / x^2x6 Algebra Graph y=5^x and y=log_5 x on a sheet of paper using the same set of axes Use the graph to describe the domain and range of each function If plugging in zero forced you to divide by zero or put a negative into a square root, those would be outside the domain of acceptable #x# values In the equation #y=2x^28#, you are neither dividing by an #x# term, nor are you dealing with #x# values under a radical sign Therefore, the domain includes all real numbersExample 2 Find the vertical and horizontal asymptotes of the graph of f(x) = 4x2 x2 8 Solution The vertical asymptotes will occur at those values of x for which the denominator is equal to zero x2 8 = 0 x2 = 8 x = p 8 Since p 8 is not a real number, the graph will have no vertical asymptotes



2
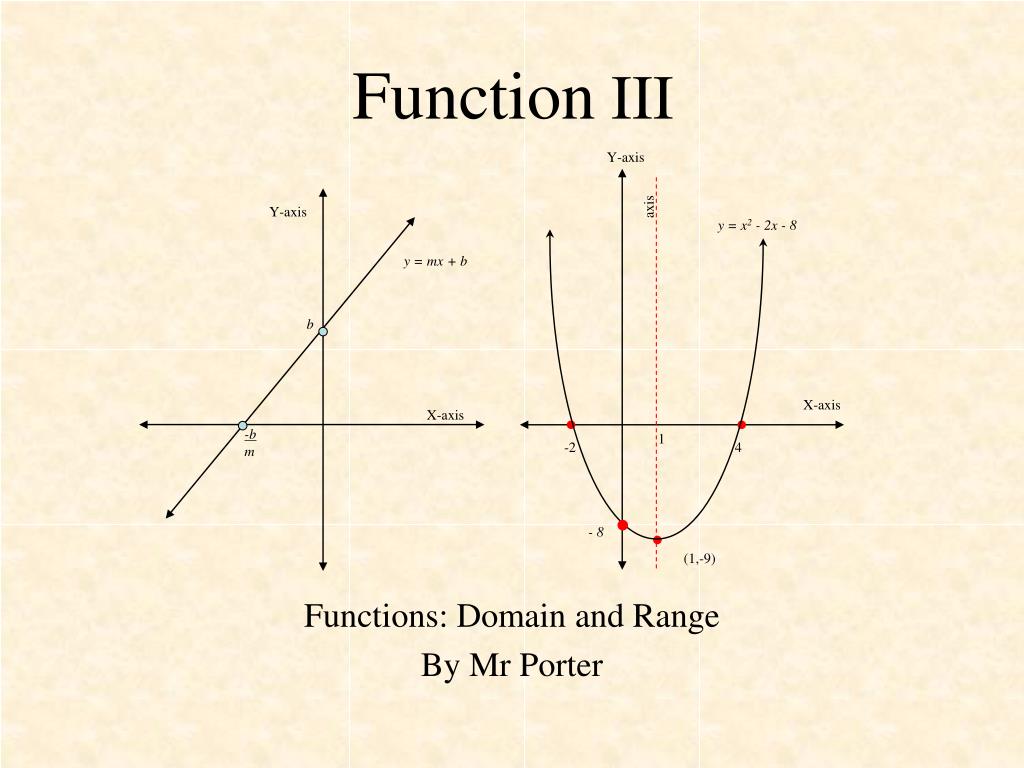



Ppt Function Iii Powerpoint Presentation Free Download Id



Amchsprecalpap Weebly Com
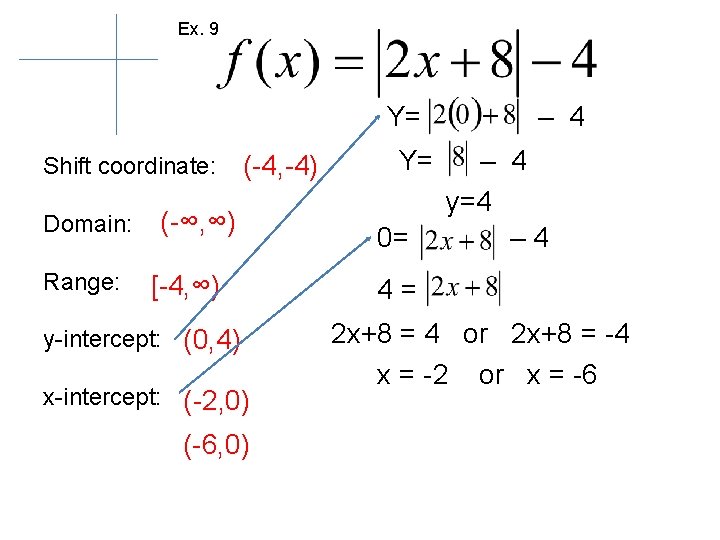



Day 5 Book Section 7 8 Review Parent



Solving Equations Algebraically




F X 3x 2x 8 Find Domain And Range Brainly In



C3 Functions




Identify Quad Parts Projectile Motion Pdf Physics Mechanics




Instantcert Credit College Algebra Lesson 22




2 5 Quadratic Functions Functions Siyavula




Graphing With A Domain 8th Grade Math 13 Homeworkhelp



2




2x 8 The Rational Function F X X2 7x 12 Has A Horizontal Asymptote At Y Homeworklib



Solved F X X 2 2x 8 Find The X Intercept S Y Intercept S Domain And Range Course Hero



Given The Quadratic Equation Y X 2 6x 5 Find The Vertex Axis Of Symmetry Intercepts Domain Range Interval Where The Function Is Increasing And Enotes Com
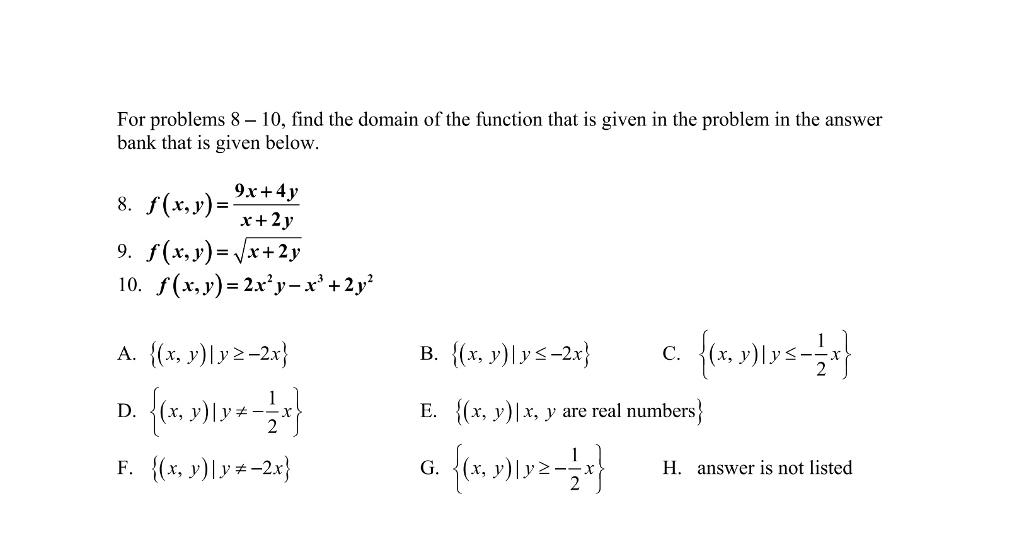



Solved Find The Domain Of The Functions Given In The Problem Chegg Com




Domain Range And Codomain



2



2




How To Graph Y Sqrt X Video Lesson Transcript Study Com



2
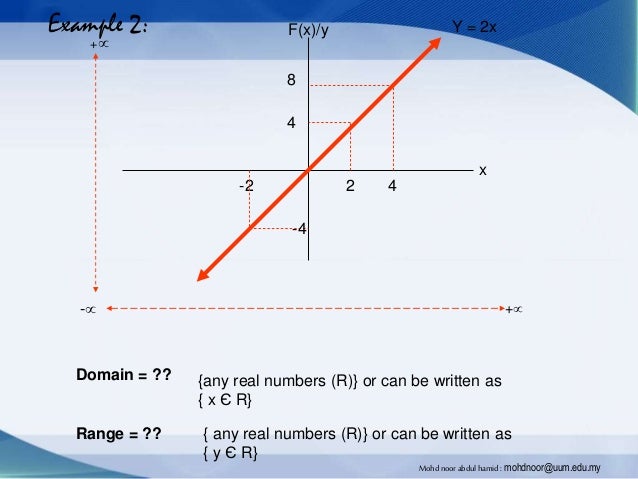



Introduction To Function Domain And Range Mohd Noor




Domain And Range Of 1 X 2 2x 8 Youtube



Domain And Range Of Quadratic Functions Video Khan Academy




Tex F X Frac X 2 4x 4 X 2 2x 8 Tex Domain V A Roots Y Int H A Holes O A Also Brainly Com




Graphing Parabolas




Restrict The Domain To Find The Inverse Of A Polynomial Function College Algebra



9y4 X Domain N 235 10ydfrac See How To Solve It At Qanda



Newpaltz K12 Ny Us




Discontinuity Calculator Wolfram Alpha




Solved The Following Function Is Given Answer The Following Chegg Com




Oneclass Sketch The Graph Of Y X 8 2x 22 2x Given That Cm 3 Is The Volume Of A Cuboid With H



Domain And Range Examples Domain And Range Of Functions



Domain And Range Of Exponential And Logarithmic Functions




7 2 Abs Value Function




View Question Domain Of 2x 8 If X 1
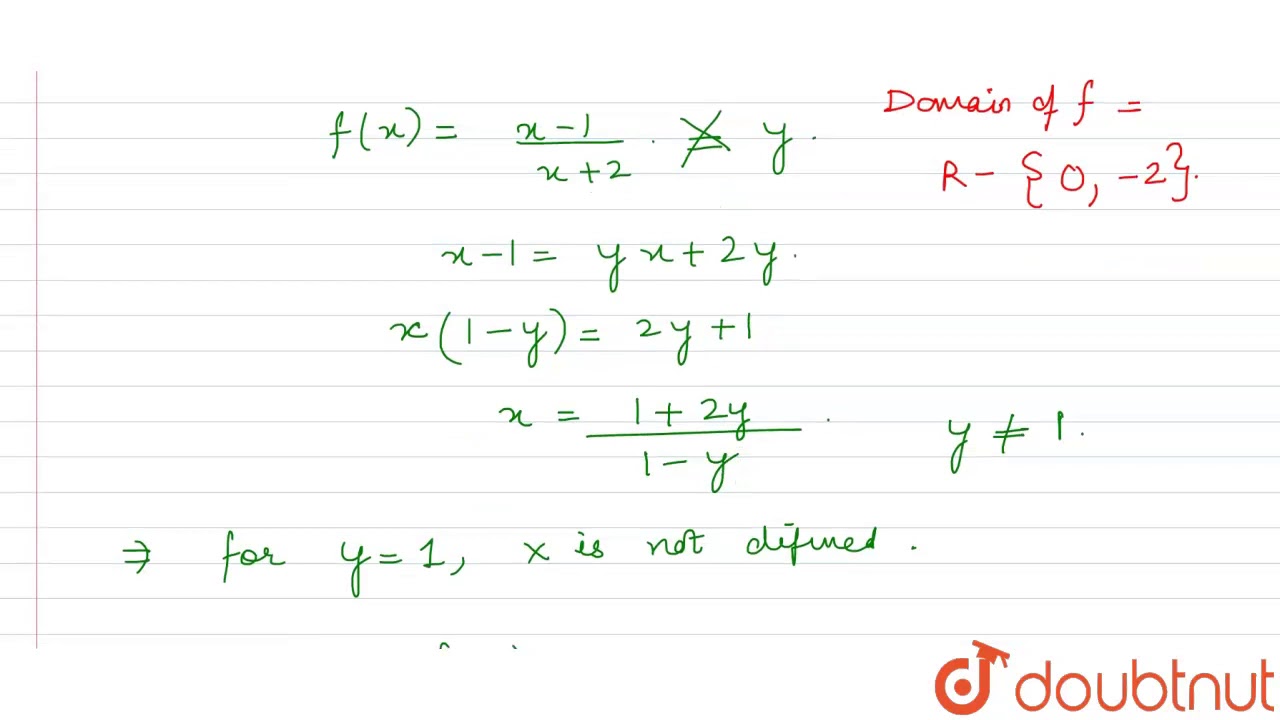



If F X X 2 X X 2 2x Then Find The Domain And Range Of F Show That F Is One One Youtube



4 13 Set Domain Graphing Calculator By Mathlab User Manual



Fkdmjqa6dd3gbm




6 Ways To Find The Domain Of A Function Wikihow




Domain Of Rational Function Assignment



2
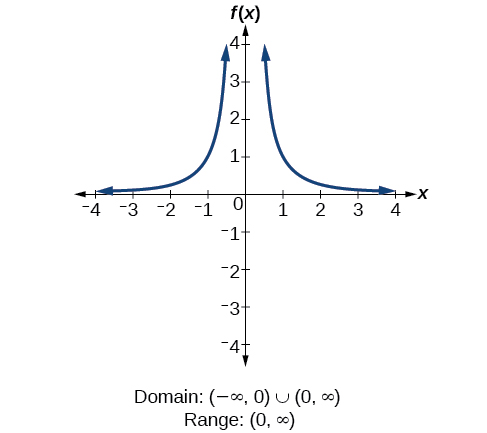



3 3 Domain And Range Mathematics Libretexts



Ppt Function Iii Powerpoint Presentation Free Download Id



1 Solve For X 1 2 2x 23 X 2 Graph The Functio Gauthmath



2



2




Which Model Shows The Correct Factorization Of X2 2x 8 Knowme Live




Redefining The Specificity Of Phosphoinositide Binding By Human Ph Domain Containing Proteins Nature Communications



For F X X 2 6x 8 Determine The Vertex Axis Of Symmetry Intercepts Domain Range Intervals Where The Function Is Increasing And Decreasing And Enotes Com
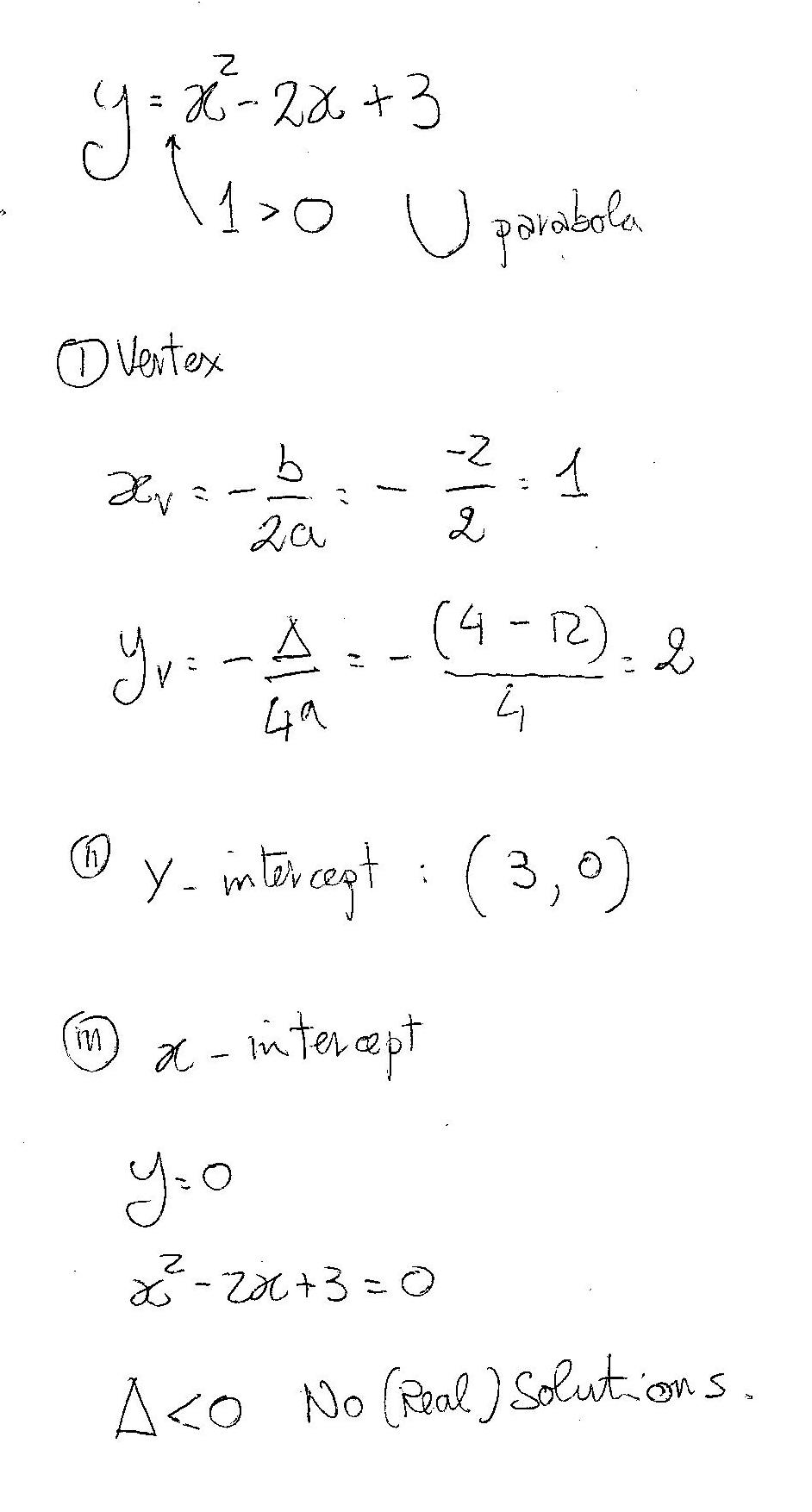



How Do You Graph Y X 2 2x 3 Socratic
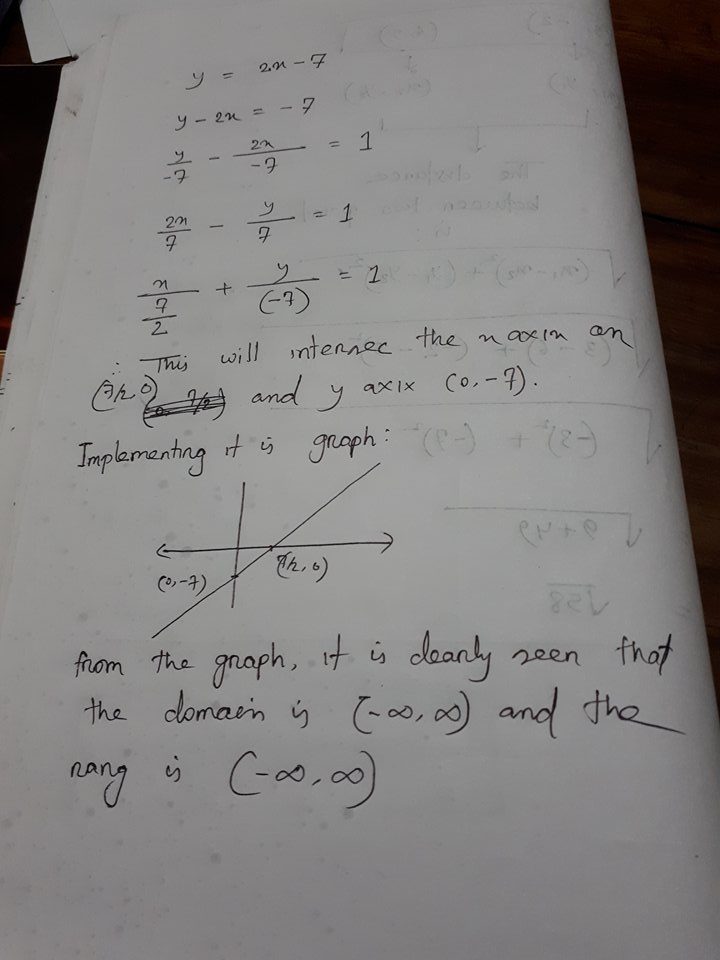



What Is The Domain And Range Of Y 2x 7 Socratic



1 Solve X2 2x 24 0 Answer 4 2 Solve 8 3x 5 Ppt Download



2




Graph F X X 2 2x 8 Youtube



What Are The Domain And Range For 1 Y X 3 And 2 Y 16 X 2 Quora



Power Function Properties Graphs Applications




Find The Domain And Range Function F X X 2 3x 2 X 2 4x 3



X2 2x 8



2



2



How To Find The Domain And Range From Y X 2 2x 8 Quora



How To Find The Domain And Range From Y X 2 2x 8 Quora



2




Find The Domain And Range Of The Function F X X 2 1 X 2




Sketch The Graph Of Y X 8 2x 22 2x Given That Y Is The Volume In Cm 3 Of A Cuboid With Height X Cm Length 22 2x Cm And




Functions Domain And Range By Mr Porter Ppt Download



Vandyke Beds Sch Uk



1



Math Colorado Edu



2



How To Find The Domain Of A Function Video Khan Academy



Solution For The Quadratic Function F X X 2 2x 8 Find A The Axis Of Symmetry And The Vertex B The X Intercepts And Y Intercepts C The Domain And Range D



2



Solved Find The Value For The Function X2 8 1 Find Ffx1 Chegg Com



2



0 件のコメント:
コメントを投稿